40th IMO shortlist 1999/G7 solution

40th IMO 1999 shortlist

Problem G7

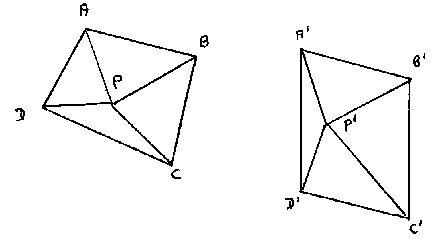
P is a point inside the convex quadrilateral ABCD such that PA = PC, ∠APB = ∠PAD + ∠PCD, and ∠CPD = ∠PCB + ∠PAB. Show that AB·PC = BC·PD and CD·PA = DA·PB.

Solution

Take another triangle A'P'B' congruent to APB. Since PA = PC, we may now take the point D' so that A'P'D' is congruent to CPD. So, in particular, PA = PC = P'A', PD = P'D'. Take the point C' so that B'P'C' is similar to APD. Now P'C'/P'D' = (P'C'/P'B')(P'B'/P'D') = (PD/PA)(PB/PD) = PB/PA = PB/PC. But ∠C'P'D' = ∠BPC (angle C'P'D' = 360^o - ∠A'P'B' - ∠B'P'C' - ∠D'P'A' = 360^o - ∠APB - ∠DPA - ∠CPD). So P'C'D' and PBC are similar.

Now ∠B'A'D' + ∠A'D'C' = (∠B'A'P' + ∠P'A'D') + (∠A'D'P' + ∠P'D'C') = ∠BAP + (∠'A'D' + ∠A'D'P') + ∠PCB = ∠BAP + (180^o - ∠A'P'D') + ∠PCB = ∠BAP + (180^o - ∠CPD) + ∠PCB = 180^o (by the relation given in the question). So A'B' is parallel to C'D'.

Similarly, ∠D'A'B' + ∠A'B'C' = (∠D'A'P' + ∠P'A'B') + (∠A'B'P' + ∠P'B'C') = ∠PCD + (∠P'A'B' + ∠A'B'P') + ∠PAD = ∠PCD + (180^o - ∠A'P'B') + ∠PAD = ∠PCD + (180^o - ∠APB) + ∠PAD = 180^o (by the other angle relation given in the question). So A'D' is parallel to B'C'.

So A'B'C'D' is a parallelogram. Hence A'B' = C'D', so AB = BC·P'D'/PC (using A'B' = AB and PBC and P'C'D' similar) = BC·PD/PC, which is the first required equation. Similarly, A'D' = B'C', so CD = AD·P'B'/PA = AD·PB/PA, which is the second required equation.

40th IMO shortlist 1999