30th IMO 1989 shortlist

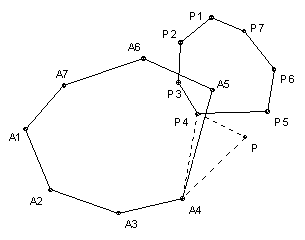
Given a convex n-gon A₁A₂ ... A_n with area A and a point P, we rotate P through an angle x about A_i to get the point P_i. Find the area of the polygon P₁P₂ ... P_n.

Solution

Consider the triangle PA_iP_i. The angle A_iPP_i is 90^o - x/2, which is independent of i, and the ratio PP_i/PA_i = 2 sin x/2, which is also independent of i. So we can obtain P_i by rotating A_i through an angle 90^o - x/2 about P and then expanding by a factor 2. Thus the area of the polygon P₁P₂ ... P_n is 4 sin²(x/2) times the area of the polygon A₁A₂ ... A_n.

30th IMO shortlist 1989

© John Scholes
jscholes@kalva.demon.co.uk
18 Dec 2002
Last corrected/updated 28 Dec 02