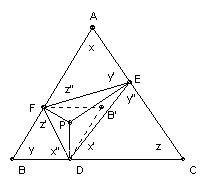

P is a point inside acute-angled the triangle ABC. The perpendiculars from P meet the sides BC, CA, AB at D, E, F, respectively. Show that P is the circumcenter iff each of the triangles AEF, BDF, CDE has perimeter not exceeding that of DEF.
Solution
One way is obvious: if P is the circumcenter, then D, E, F are the midpoints of the sides and so AEF, BDF, CDE, DEF all have the same area. So assume area AEF ≤ area DEF, area BDF ≤ area DEF and area CDE ≤ area DEF, but P is not the circumcenter. We will obtain a contradiction.

Let the angles in the triangles be as shown in the diagram (there was not room to mark y" = angle DEC.) Take B' so that BDB'F is a parallelogram. Then area BDF = area B'DF, so E cannot lie inside the triangle B'DF (except possibly at B' itself), because then we would have area DEF < area BDF. Suppose E = B'. Then EF is parallel to BC, and DE is parallel to AB. Hence PD is perpendicular to EF, and PF is perpendicular to DE. Hence P is the orthocenter of DEF and so PE is perpendicular to DF, so DF is parallel to AC. Hence BDEF and AFDE are parallelograms, so BF = DE = AF, in other words F is the midpoint of AB. Similarly, D and E are also midpoints. Hence P is the circumcenter. Contradiction.
So we may assume that E is not any point of B'DF. Hence either x' < y or z" < y. Similarly, y' < z or x" < z, and z' < x or y" < x. Without loss of generality we may assume y' < z (there is symmetry between y' and y"). So looking at triangle AEF, z" > y. Hence x' < y. So looking at CDE, y" > x. Hence z' < x. Looking at BDF, x" > z. So x' < y < z", y' < z < x", and z' < x < y".
Now AEPF is cyclic, so ∠AEF = ∠APF, so AP = PF/cos y'. Similarly, BDPF is cyclic, so ∠BDF = ∠BPF, so BP= PF/cos x" > PF/cos y' = AP. Similarly, CP > BP and AP > CP. Contradiction.
![]()
© John Scholes
jscholes@kalva.demon.co.uk
14 Oct 2002