5th Putnam 1942/B5 solution

5th Putnam 1942

Problem B5

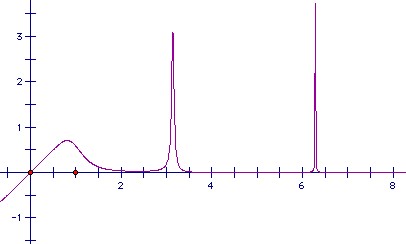
Let f(x) = x/(1 + x⁶sin²x). Sketch the curve y = f(x) and show that ∫₀^∞ f(x) dx exists.

Solution

Obviously f(x) is positive for positive x. But it has an infinite number of spikes at x = nπ. The spike at nπ is height nπ, so we have to show that the integral is bounded above.

We have sin x > ½x near x = 0 (certainly for x < π/3). So |sin x| > 1/(nπ)^k except possibly for |x| < 2/(nπ)^k. Let I_n be the interval centered on nπ width 4/(nπ)^k. For x ∈ I_n we have f(x) < 2nπ, so the integral of f(x) over the interval is less than 8/(nπ)^k-1. The total integral over all such intervals is bounded provided that k > 2. Outside such intervals, x⁶ sin²x > ½ x⁶/x^2k, so f(x) < 2/x^2k-5. Hence the interval of f(x) over 0 to ∞ excluding the intervals I_n is bounded provided k > 5/2. By taking k = 2 1/4, for example, we get that the whole integral is bounded.

5th Putnam 1942